Exploring The Fascinating World Of The Seven Sided Regular Polygon

Have you ever wondered about the unique properties of a seven sided regular polygon? Known as a heptagon, this geometric shape is a fascinating example of symmetry and mathematical precision. With its seven equal sides and angles, the seven sided regular polygon holds a special place in geometry, offering insights into both theoretical mathematics and practical applications. Whether you're a student, a math enthusiast, or simply curious about shapes, understanding this polygon can deepen your appreciation for the beauty of geometry.

The seven sided regular polygon, or heptagon, is not just a theoretical construct; it has practical implications in architecture, design, and even nature. From ancient structures to modern art, the heptagon's symmetry and balance have inspired countless creations. Its unique properties make it an intriguing subject for exploration, as it bridges the gap between simple polygons like triangles and more complex ones like dodecagons.

What makes the seven sided regular polygon so captivating? The answer lies in its balance of simplicity and complexity. Unlike polygons with fewer sides, the heptagon challenges our understanding of angles and proportions while remaining accessible to those with a basic knowledge of geometry. This article will guide you through the intricacies of the seven sided regular polygon, answering your questions and providing valuable insights into its applications and significance.

Read also:

Discovering Ulie Graham A Comprehensive Guide To His Life And Achievements

What is a Seven Sided Regular Polygon?

A seven sided regular polygon, commonly referred to as a heptagon, is a polygon with seven equal sides and seven equal angles. Each internal angle of a regular heptagon measures approximately 128.57 degrees, making it a unique shape in geometry. Unlike simpler polygons like triangles or squares, the heptagon's complexity lies in its symmetry and the precision required to construct it.

To better understand the heptagon, let's break down its defining characteristics:

• Equal Sides: All seven sides of the polygon are of the same length.
• Equal Angles: Each internal angle is congruent, ensuring perfect symmetry.
• Sum of Angles: The sum of the internal angles of a heptagon is 900 degrees.

The heptagon's unique properties make it a popular subject in both theoretical and applied mathematics. Its symmetry and balance are often used as a foundation for exploring more complex geometric shapes.

Why is the Seven Sided Regular Polygon So Special?

The seven sided regular polygon stands out among other polygons due to its unique combination of simplicity and complexity. While polygons with fewer sides, like triangles and quadrilaterals, are easier to construct and analyze, the heptagon offers a greater challenge. This challenge, however, is what makes it so intriguing to mathematicians and designers alike.

One reason the heptagon is special is its inability to be constructed perfectly using only a compass and straightedge. Unlike polygons with sides that are powers of two (e.g., squares, octagons), the heptagon requires more advanced techniques for precise construction. This limitation has fascinated mathematicians for centuries, leading to the development of innovative geometric methods.

Additionally, the heptagon's symmetry has inspired countless works of art and architecture. Its balanced proportions make it an ideal shape for creating visually appealing designs. From ancient mosaics to modern logos, the seven sided regular polygon continues to captivate creators and audiences alike.

Read also:

Exploring The Life And Achievements Of Aneesha Joshi

How to Draw a Seven Sided Regular Polygon?

Drawing a seven sided regular polygon may seem daunting at first, but with the right tools and techniques, it can be a rewarding experience. Here’s a step-by-step guide to help you create your own heptagon:

1. Gather Your Tools: You’ll need a compass, a ruler, and a protractor.
2. Draw a Circle: Use the compass to draw a perfect circle on your paper.
3. Divide the Circle: Using the protractor, divide the circle into seven equal sections by marking points at 51.43-degree intervals (360 degrees divided by 7).
4. Connect the Points: Use the ruler to connect the marked points, forming the sides of the heptagon.

While this method provides a close approximation, achieving absolute precision requires more advanced mathematical tools. Nevertheless, this exercise offers a hands-on way to explore the properties of the seven sided regular polygon.

What Are the Properties of a Seven Sided Regular Polygon?

The seven sided regular polygon boasts several unique properties that set it apart from other polygons. Understanding these properties is key to appreciating its role in geometry and design:

• Internal Angles: Each internal angle of a regular heptagon measures approximately 128.57 degrees.
• Exterior Angles: The exterior angles of a heptagon measure approximately 51.43 degrees.
• Diagonals: A heptagon has 14 diagonals, which can be calculated using the formula n(n-3)/2, where n is the number of sides.

These properties make the heptagon a versatile shape for various applications, from architectural design to artistic compositions.

Applications of the Seven Sided Regular Polygon

The seven sided regular polygon finds applications in a variety of fields, showcasing its versatility and utility. From architecture to nature, the heptagon's unique properties make it a valuable tool for designers and scientists alike.

How Does the Seven Sided Regular Polygon Appear in Nature?

While not as common as hexagons, the seven sided regular polygon can still be found in nature. Certain crystals and molecular structures exhibit heptagonal symmetry, highlighting the polygon's role in natural geometry. Observing these natural occurrences can deepen our understanding of the heptagon's significance.

Famous Structures Inspired by Seven Sided Regular Polygons

Throughout history, architects and designers have drawn inspiration from the heptagon. From ancient temples to modern skyscrapers, the seven sided regular polygon has been used to create visually striking and structurally sound buildings.

Common Misconceptions About Seven Sided Regular Polygons

Despite its fascinating properties, the heptagon is often misunderstood. One common misconception is that it can be constructed perfectly using only a compass and straightedge. This myth has been debunked by mathematicians, who have shown that achieving perfect precision requires more advanced tools.

How Can You Use Seven Sided Regular Polygons in Design?

Designers often use the heptagon to create balanced and visually appealing compositions. Its symmetry and unique proportions make it an ideal choice for logos, patterns, and architectural elements.

Frequently Asked Questions About Seven Sided Regular Polygons

What is the difference between a regular and irregular heptagon? A regular heptagon has equal sides and angles, while an irregular heptagon does not.

Can a heptagon tessellate a plane? No, a regular heptagon cannot tessellate a plane because its angles do not divide evenly into 360 degrees.

Why is the heptagon important in mathematics? The heptagon's unique properties make it a valuable tool for exploring geometric principles and solving complex problems.

How does the heptagon compare to other polygons? While simpler polygons like triangles and squares are easier to construct, the heptagon offers a greater challenge and a deeper understanding of geometric symmetry.

Exploring The World Of Vanillagidt.com: Your Ultimate Guide To Unique Experiences
Understanding Acres In A Section: A Comprehensive Guide
Unlocking The Mysteries Of Numerology 1111: A Journey Into Spiritual Awakening

• Viral Insta Mms Video The Ultimate Guide To Understanding Its Impact

Details

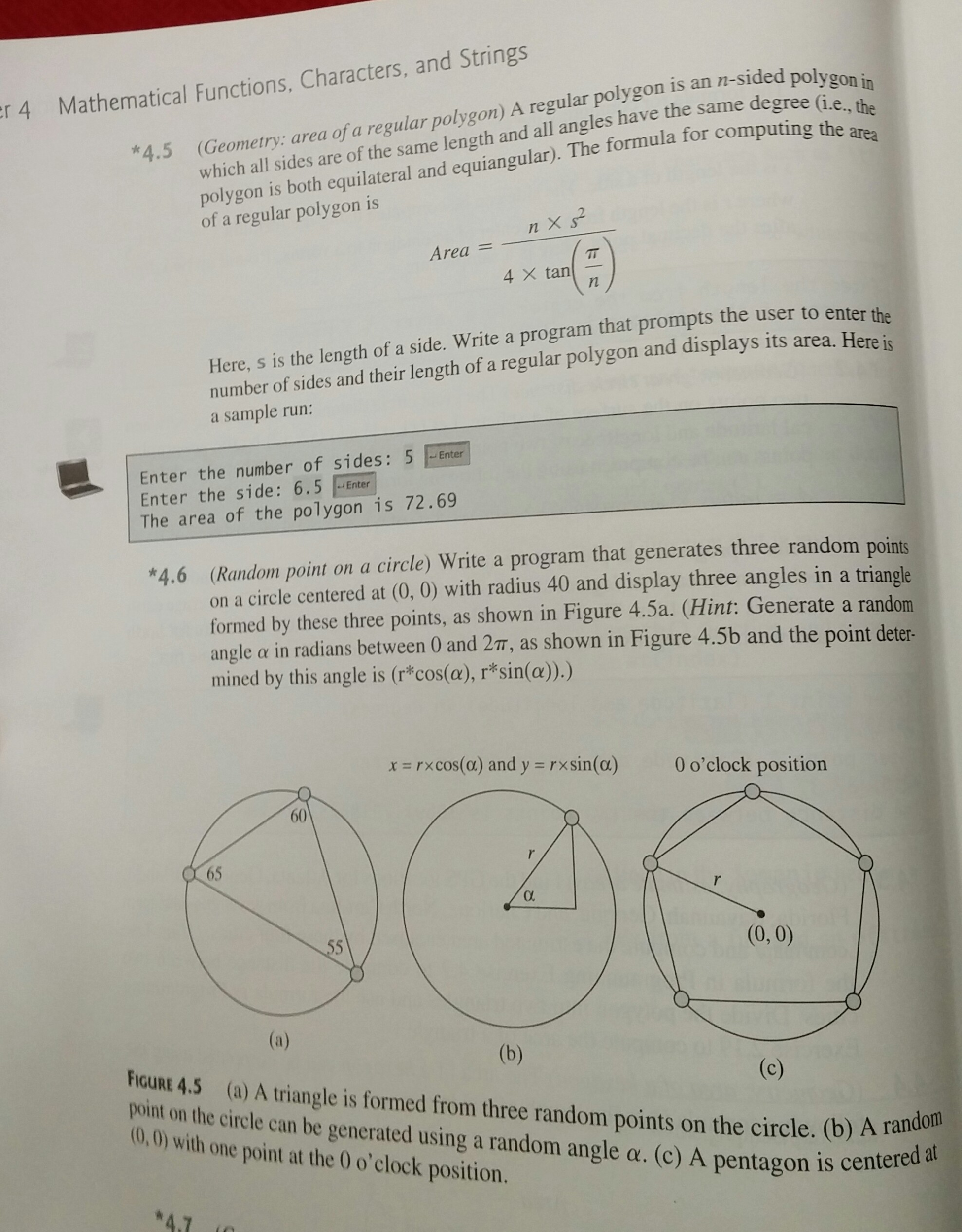
Solved A regular polygon is an nsided polygon in which all

Details

34+ A 9 Sided Polygon Pictures Nosha